If a crewed spacecraft could be built to travel at or near the speed of light, how long would it take to achieve that speed without crushing the human occupants?
Lawrence Schwab
Phoenix, Arizona
Despite what science fiction may suggest, humanity will never be able to travel at the speed of light. That said, we could theoretically get close. To cause the fewest problems for our human occupants, it would best to maintain the acceleration our body is familiar with: Earth’s gravitational pull. At the surface of our planet, that is 32.2 feet/second2 (9.8 meters/second2). Accelerating at that rate, from the perspective of the traveler it would take 2.65 years to reach 99 percent the speed of light.
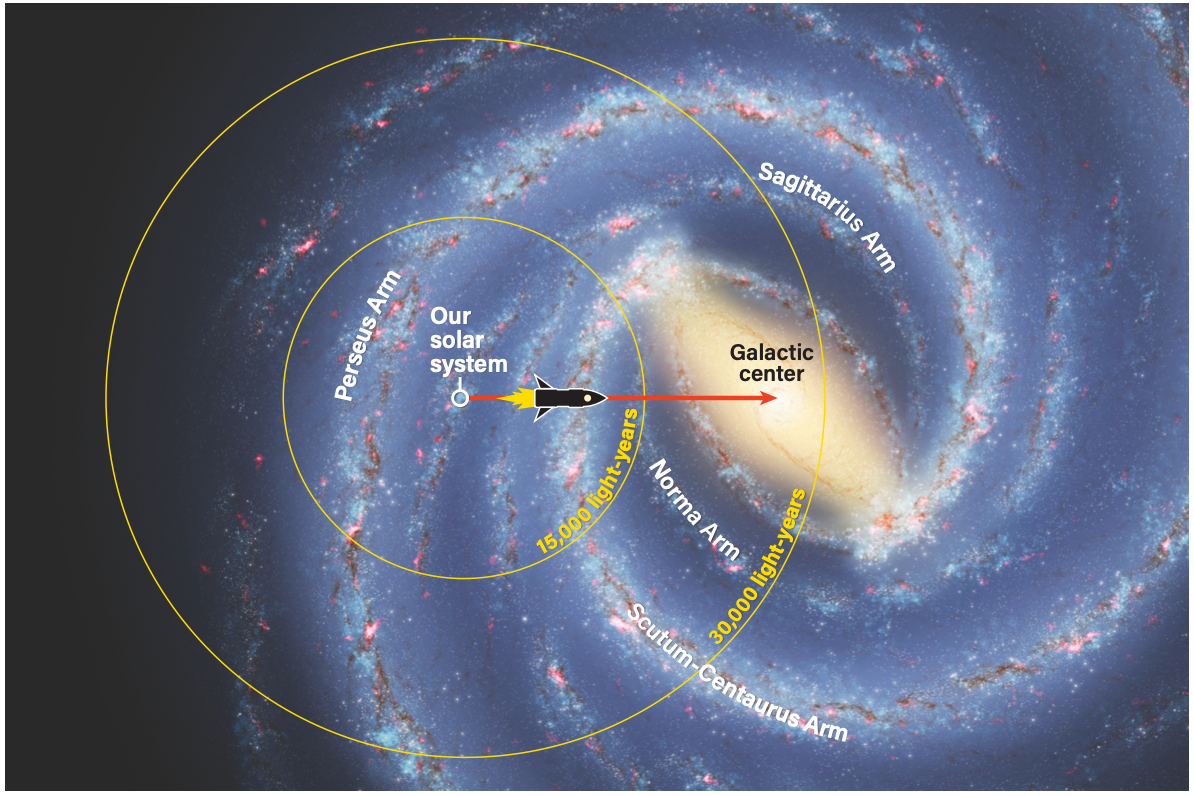
To better appreciate the result, consider that such a traveler wants to reach the center of the Milky Way. That’s nearly 30,000 light-years distant. For half that distance, they’ll want to accelerate at 32.2 ft/s2 (9.8 m/s2) and then decelerate by that same amount for the remaining half of the journey. At that acceleration, it would take the traveler around 20 years to get there — 40 years for a round trip.
But some weird things happen to time when moving close to the speed of light.
While only a few decades have passed for the traveler, significantly more time will have passed on Earth. Let’s assume the impossible: The traveler manages to travel at the speed of light the entire way. In this case, nearly 30,000 years will have passed on Earth by the time they reach the center of the galaxy. For the round-trip journey, 60,000 years will have gone by at the end from Earth’s perspective.
Needless to say, the traveler’s friends at the time of departure would no longer be around when they return. This difference in observed time, or time dilation, is a consequence of Einstein’s special relativity and illustrates his famous twin paradox.
Stuart L. Shapiro
Professor of Physics and Astronomy, University of Illinois, Urbana-Champaign, Illinois









